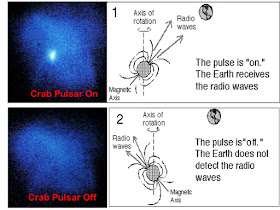
A science blog designed for community education. Climate Change, Sustainable Development, Lifestyle and Health are recurring themes. Biographies of famous scientists like Curie, Watt, Fleming, Einstein, Galileo etc. are available. Biomedical Imaging, Nanotechnology,Theory of Relativity, Physics of the Nucleus, Making of the Atomic Bomb, Plate Tectonics and many other subjects are discussed in detail without the use of higher maths. For further information - Contact ektalks@yahoo.co.uk
Pages
▼
Sunday, 13 December 2015
Paris Climate Agreement - Too Little Too Late - Nations buy time to do nothing for at least 5 more years
I had made a resolution that I shall not comment on the Paris Climate Change Agreement - this was not to be; and I feel compelled to say my bit on an agreement that is not quantitative enough but a restatement of 'good intentions' albeit more cleverly drafted.
CO2 levels have risen from about 300 ppm to 400 ppm since the Industrial Revolution - a good part coming from human activities of burning fossil fuel and deforestation. Carbon isotope analysis has confirmed this beyond any doubt whatsoever. People who claim otherwise are only being silly. The average Global temperature has already risen by 0.8C and could rise by a total of 2.7C even if all the good intentions of the Paris agreement are delivered.
It will be good to watch what the oil company share price does over the next few months - I bet that it will not crash as it should if the Paris agreement has any meaning and fossil fuel consumption is reduced. The share price will vindicate my belief that nations of the world will not implement what they have promised in Paris yesterday.
The basic problem is money - Bloomberg analysis says it will cost 16500 Billion dollars to shift energy production from fossil to renewable etc. and the biggest developing economies of China and India will not be inclined to find big monies to correct a problem that USA and Europe caused and who also have greater resources. It really boils down to sustainability. The carbon foot print of the developed countries is far greater than the average global foot print. A large fraction of CO2 emission from China and other developing countries goes to produce and transport goods for consumption in the developed countries. We have got used to living on cheaply produced goods - no wonder inflation is so hard to materialize in spite of masses of Quantitative Easing by the central banks run by very clever, and very rich, people. We have mortgaged the welfare of the next few generations already. The climate change effects of rising sea levels, selective severe droughts and rainfalls, new diseases moving north, frequent extreme events will be just a few extra miseries they will need to cope with.
If we keep burning fossil fuels at the current level then CO2 levels will not come down - they will not be coming down in a hurry anyway as once CO2 is in the atmosphere, it will hang around there for much more than a 100 years. We are already committed. Emissions need to be cut - and cut drastically - to limit temperature increase to less than 2C.
General wisdom is to limit what one has to say to an A4 sheet of paper. I could go on for a few more pages but that will be unwise.
Take care and Bye until the next time...
Blog Contents - Who am I?
Saturday, 28 November 2015
Logarithms - Useful Background to Understand Power Laws in Biological Scaling
Biological Scaling: Importance of Size: Laws of
Physics determine how animals are formed and how they function. Animal bodies are designed to operate under the constraints set by the
laws of physics. Strength of animal bones will determine the maximum
mass it can have. How efficiently heat produced by metabolic activity
inside the body may be eliminated, how oxygen is transferred to the blood -
these processes have limits set by physics and determine the shape and size and
methods that animals use to optimize their bodies.
What is amazing is that many body functions of animals, like
metabolic activity, heart size, oxygen consumption etc. follow general laws
expressed as some power of their mass, and the
same power laws hold over a very wide size range from the tiny shrew to the
massive whale.
I intend to look at biological scaling laws.
It can be sometimes
difficult to interpret power laws as they are non-linear functions. Power laws may be expressed in an
'easier to interpret' linear form by using logarithms. Logarithms are not somethings that
everybody is totally comfortable with and a short primer on logs might be useful before discussing the subject of scaling laws in more detail.
In mathematics, we define a function as an operation that changes the value, in a well-defined way, of the quantity it operates on. For example:
square root is a function is sqrt(9) = 3
tan is a trigonometric function tan(60) = 1.732
Most frequently used functions are found as hot-buttons on your
scientific calculator.
Similarly logarithm is a function, also found as a hot-button
(log) on your calculator.
Logs are useful as they change products to sums, and divisions to differences
log (AB) = log A + log B
log (A/B) = log A – log B
For a power relation:
log (A3) = log (A.A.A) = log A + log A + log A = 3 log A
log (A3) = log (A.A.A) = log A + log A + log A = 3 log A
If
y = xn then
log
y = n log x
n does not have to be an integer. It can also be a positive or
negative number.
In the case of a power law of the form y = a xn
we have log y = log (a xn) = log a + log (xn) = log a +
n log x
You might notice that log y
= log a + n log x
is the equation of a straight line when one plots log y along the y-axis and log x along the x-axis. Thus we have converted a power relation which plots a curve, to a linear relation that will plot a straight line. Also notice that the slope of the straight line is the exponent n of the power relation and the intercept on the y-axis is log(a).
is the equation of a straight line when one plots log y along the y-axis and log x along the x-axis. Thus we have converted a power relation which plots a curve, to a linear relation that will plot a straight line. Also notice that the slope of the straight line is the exponent n of the power relation and the intercept on the y-axis is log(a).
The log functions we shall use are to the base 10. This is what the scientific calculator gives
too. This means that log10 = 1 (you can check it on your calculator)
To understand it better:
Suppose you take log of number 2.
It will be a number p such that 2 = 10p
Taking logs gives us log 2 = log (10p) = p log10 = p since log10 = 1
Using your calculator you will find that p = 0.30103
In the following, I show how logs change power relations to linear relations: The XCEL worksheet shows what is plotted in the two graphs that follow:
(please click on the slide to see its bigger image)
Blog Contents - Who am I?